Hi there. Hope you and yours are well in these difficult times!
This is just a very short announcement that I’ll be doing an on-line version of my Excel VBA Masterclass.
The training is scheduled for May 18, 20, 26, 28, June 2, 4 and I’ll be using Microsoft Teams to deliver it to your homes!
Regards,
Jan Karel Pieterse
Add-ins have worksheets. You just can’t see them. But you can store information on them and it’s a good way to store settings, preferences, and other data. When you want to get to that data, you can go to the Properties for ThisWorkbook and change the IsAddin property to False. Now you can see the worksheets and change the data if necessary.
When you’re done, go back to the VBE and change the IsAddin property back to True before you save your changes. Don’t forget that part; it’s important.
I have a list of vendor codes stored on a worksheet in an addin. I need to see the list, but not change it. I didn’t want to go through all the IsAddin rigmarole, so I did this in the Immediate Window.
|
1 |
wshvendors.ListObjects(1).ListColumns(1).DataBodyRange.value |
That part returns a two-dimensional array of all the values in the first column
|
1 |
application.transpose(...) |
That turns a two-dimensional array into a one-dimensional array.
|
1 |
?join(...,",") |
That turns an array into a string with commas between the values. In retrospect, I should have used
|
1 |
?join(application.transpose(wshvendors.ListObjects(1).ListColumns(1).DataBodyRange.value),vbnewline) |
to get each code on its own line. Here’s a way to put it into a range, if that’s where you’re going with it anyway.
|
1 |
wshvendors.ListObjects(1).ListColumns(1).DataBodyRange.Copy workbooks.Add.Worksheets(1).cells(1,1) |
I read Charles William’s MaxMinFair algorithm and I didn’t like his approach. That’s typical. I’ll read somebody’s code and think “They’re making that too hard”. Then I’ll set about rewriting it. In this case, as in most cases, it turns out that it is that hard, but I wasn’t going to convince myself until I tried it. I ended up with a different approach that’s not shorter, not easier to read, and not easier to follow. Oh well, here it is anyway.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 |
Function MaxMinFairDK(Supply As Double, Demands As Variant) As Variant Dim dPrior As Double Dim vaReturn As Variant Dim dAvailable As Double Dim i As Long, j As Long Dim dTemp As Double Dim wf As WorksheetFunction On Error GoTo ErrHandler Set wf = Application.WorksheetFunction If IsObject(Demands) Then Demands = Demands.Value2 'make range array dAvailable = Abs(Supply) 'ignore negative supplies If Not IsArray(Demands) Then 'One demand = min of supply or demand MaxMinFairDK = Array(dAvailable, Demands)(Abs(dAvailable > Demands)) Else 'Excel returns NA when you use too many columns If UBound(Demands, 2) > 1 Then Err.Raise xlErrNA 'Assume everybody gets everything they want ReDim vaReturn(LBound(Demands, 1) To UBound(Demands, 1), 1 To 1) vaReturn = Demands For i = UBound(Demands, 1) To LBound(Demands, 1) Step -1 'If there's enough, do nothing except reduce what's available If dAvailable / i > (wf.Large(Demands, i) - dPrior) Then dAvailable = dAvailable - ((wf.Large(Demands, i) - dPrior) * i) dPrior = wf.Large(Demands, i) Else 'Once there's not enough, everyone splits what's left For j = LBound(Demands, 1) To UBound(Demands, 1) If Demands(j, 1) > dPrior Then vaReturn(j, 1) = dPrior + (dAvailable / i) End If Next j Exit For End If Next i MaxMinFairDK = vaReturn End If ErrExit: Exit Function ErrHandler: MaxMinFairDK = CVErr(Err.Number) Resume ErrExit End Function |
In Charles’s implementation, he allocates an equal amount of the supply to each node, then takes back what that node didn’t need and puts it back in the available pool. When I was looking at the results, I was thinking that the smallest n nodes simply get their demand and only when there’s not enough to go around do we need to do something different than allocate the full demand.
In my implementation, I start by giving everyone what they demand. Then I start with the smallest demand, and if I can accommodate that amount for everyone, I just reduce the amount available and move to the second smallest demand. At some point (the sixth smallest demand in Charles’s data) I can’t meet that demand and still give everyone an equal share. At that point, I give anyone who hasn’t had their demand met an equal amount – the amount that’s already been distributed plus an equal share of what’s left.
| Rank | Demand | Incremental Demand | Allocated | Remaining |
|---|---|---|---|---|
| 18.30 | ||||
| 7 | 0.70 | 0.70 | 4.90 | 13.40 |
| 6 | 1.00 | 0.30 | 1.80 | 11.60 |
| 5 | 1.30 | 0.30 | 1.50 | 10.10 |
| 4 | 2.00 | 0.70 | 2.80 | 7.30 |
| 3 | 3.50 | 1.50 | 4.50 | 2.80 |
| 2 | 7.40 | 3.90 | 7.80 | (5.00) |
| 1 | 10.00 | 2.60 | 2.60 | (7.60) |
In the first iteration, I hand out 0.70 to everyone because I have enough supply to do that. In the second iteration, I had out the differential, 0.30, to everyone who’s left because I have enough supply remaining. When I get to #2, I can’t hand out 3.90 to the remaining two nodes because I don’t have enough supply. I’ve allocated up to 3.5 to anyone who’s demanded it, so the last two get the 3.5 plus half of the 2.8 that remains.
Although I didn’t accomplish anything, it was still a fun exercise.
The Join function takes an array and smushes it together into a String. I love the Join function. The only thing I don’t like about it is when I forget that it doesn’t work on 2d arrays. Join only works with 1-dimensional arrays. The last time my memory failed me, I decided to write my own. And here it is.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 |
Public Function Join2D(ByVal vArray As Variant, Optional ByVal sWordDelim As String = " ", Optional ByVal sLineDelim As String = vbNewLine) As String Dim i As Long, j As Long Dim aReturn() As String Dim aLine() As String ReDim aReturn(LBound(vArray, 1) To UBound(vArray, 1)) ReDim aLine(LBound(vArray, 2) To UBound(vArray, 2)) For i = LBound(vArray, 1) To UBound(vArray, 1) For j = LBound(vArray, 2) To UBound(vArray, 2) 'Put the current line into a 1d array aLine(j) = vArray(i, j) Next j 'Join the current line into a 1d array aReturn(i) = Join(aLine, sWordDelim) Next i Join2D = Join(aReturn, sLineDelim) End Function |
It’s pretty simple. It loops through the first dimension (the row dimension) and joins each line with sLineDelim. Inside that loop, it joins each element in the second dimension with sWordDelim. What this function doesn’t do is automatically insert itself into only the projects I want. That requires me to remember that I wrote it and where I put it. In reality, I’ll probably reinvent the wheel the next time I need it.
Here’s my extensive testing procedure.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 |
Sub TEST_Join2d() Dim a(1 To 2, 1 To 2) As String a(1, 1) = "The" a(1, 2) = "Quick" a(2, 1) = "Brown" a(2, 2) = "Fox" Debug.Print Join2D(a) Debug.Print Debug.Print Join2D(a, ",") Debug.Print Debug.Print Join2D(a, , "|") Debug.Print Debug.Print Join2D(a, ";", "||") End Sub |
Since my recent move to Digital Ocean for hosting, I’ve had to make a change to how I upload images for this blog. I used to create an FTP file and a batch file, but as far as I know that doesn’t support SFTP. I’m using WinSCP to transfer files manually and learned that it has a command line interface. I made a procedure called SendViaSCP to replace my SendViaFTP.
Public Sub SendViaSCP(vFname As Variant)
Dim aScript() As String
Dim i As Long
ReDim aScript(1 To 4 + UBound(vFname))
aScript(1) = "option batch abort"
aScript(2) = "option confirm off"
aScript(3) = "open sftp://username:password@000.000.000.000"
aScript(UBound(aScript)) = "exit"
For i = LBound(vFname) To UBound(vFname)
aScript(3 + i) = "put " & Dir(vFname(i)) & " /home/wordpress/public_html/blogpix/"
Next i
Open "winscpup.txt" For Output As #1
Print #1, Join(aScript, vbNewLine)
Close #1
Shell "winscpup.bat"
End Sub
The vFname arguments is a variant array that holds all of the files I selected from Application.GetOpenFileName. The aScript array holds three lines of setup, a line for each file, and an exit line.
The commands are joined together and written to a batch file and the batch file is run. It doesn’t solve the problem that Billkamm and Haines solved of having your username and password in a batch file, but I can live with it.
You might be wondering why I don’t just use the file upload functions in WordPress. What fun would that be?
I have a list of labels and a list of values. I also have a value from somewhere in the range of my values list.
I want to show where the value falls in the list of values with a red mark. The labels need to be proportional to their values. An XY chart seemed like the obvious answer, but would require the XY Chart Label add-in or some better charting skills on my part. Also, there may be a few of these on a sheet, so I wanted to keep it more light-weight. Sparklines move nicely with cells, so I tried that route.
First, I needed to get the labels spread proportionally across a cell. I made the cell 20 characters wide by typing '00000000000000000000, setting the font to Courier New, and resizing the column. Cell C6 shows the positions. To get the proper spread of labels, I used a UDF, shown below.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 |
Public Function PropAxis(rLabels As Range, rValues As Range) As String Dim vaLabels As Variant Dim vaValues As Variant Dim i As Long, j As Long Dim dSpan As Double Dim dIncrement As Double Dim lPosition As Long Dim aReturn(1 To 20) As String 'Put ranges in an array vaLabels = rLabels.Value vaValues = rValues.Value 'Find the span of the values range dSpan = vaValues(UBound(vaValues, 1), 1) - vaValues(LBound(vaValues, 1), 1) 'initialize the array with spaces For i = LBound(aReturn) To UBound(aReturn) aReturn(i) = Space(1) Next i 'put the first and last labels in the array aReturn(1) = vaLabels(1, 1) aReturn(UBound(aReturn)) = vaLabels(UBound(vaLabels, 1), 1) 'Put the middle labels proportionally For i = LBound(vaLabels) + 1 To UBound(vaLabels) - 1 dIncrement = (vaValues(i, 1) - vaValues(1, 1)) / dSpan dIncrement = dIncrement * (UBound(aReturn) - 1) lPosition = Round(dIncrement, 0) 'If they're too close, just move one over If aReturn(lPosition) <> Space(1) And lPosition < UBound(aReturn) Then lPosition = lPosition + 1 End If aReturn(lPosition) = vaLabels(i, 1) Next i PropAxis = Join(aReturn, vbNullString) End Function |
The values still have to be reasonably spread out or this will overwrite some of them. But it worked for my purposes. The formula =PropAxis(F3:F8,G3:G8) is in C3.
Next I set up the range I2:AB3 to feed the sparkline. Row 2 represents the 20 characters in the cell. Row three should be all zeros except one, which will be a 1. Then a win/loss sparkline will show the one ‘win’ as a mark.
In H2, I calculate which character gets the win. Here’s how that formula progressed:
=ROUND((G2-G3)/(G8-G3)*20,0)
That figures where the value is in the list of values as a percentage and multiplies by the number of characters in the cell (20). I wanted to protect against a value that was not in the range, so I modified it to this:
=MIN(MAX(ROUND((G2-G3)/(G8-G3)*20,0),1),20)
Now it will never be less than 1 or greater than 20. Because of rounding, the mark may be one character off of an exact match. That’s probably not a problem – it’s obviously not supposed to be hyper-accurate since I’m only using 20 characters – but it just doesn’t look right. So I handle exact matches as special cases.
=IF(ISNA(MATCH(G2,G3:G8,FALSE)),MIN(MAX(ROUND((G2-G3)/(G8-G3)*20,0),1),20),FIND(INDEX(F3:F8,MATCH(G2,G3:G8,FALSE),1),C3))
If there isn’t an exact match, find the percentage and get close. If it is an exact match, find the character’s position in the string and put the mark there. Now in Row 3 of my sparkline data range, I use this number to find the ‘win’.
=IF($H$2=I2,1,0)
I thought this whole process would be easier with sparklines. But it’s not really any more light-weight than just a chart. Anybody else want to take a crack at it?
You can download SparklineProportion.zip
I’ve restored a few posts in the last few months that were lost. I didn’t restore any of the comments. Honestly, I should have but I didn’t even think about it. But when I went to restore the In Cell Charting post, I noticed there were 85 comments. That seemed worth my while.
First I set a reference to Microsoft XML, v6.0 and Microsoft HTML Object Library. Here’s the main procedure.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 |
Public Sub CreateCommentSQL() Dim xHttp As MSXML2.XMLHTTP Dim hDoc As MSHTML.HTMLDocument Dim hOLComments As MSHTML.HTMLOListElement Dim hLIComments As MSHTML.IHTMLElementCollection Dim hLIComment As MSHTML.HTMLLIElement Dim clsComments As CComments Dim clsComment As CComment 'Go get the lost comments from the wayback machine Set xHttp = New MSXML2.XMLHTTP xHttp.Open "GET", "http://web.archive.org/web/20100418043617/http://www.dailydoseofexcel.com/archives/2006/02/05/in-cell-charting/" xHttp.send 'Wait until the page loads Do: DoEvents: Loop Until xHttp.readyState = 4 Set clsComments = New CComments 'Load the document Set hDoc = New MSHTML.HTMLDocument hDoc.body.innerHTML = xHttp.responseText 'The ordered list has an id of "comments" Set hOLComments = hDoc.getElementsByName("comments")(0) 'Get all the listindex elements Set hLIComments = hOLComments.getElementsByTagName("li") For Each hLIComment In hLIComments Set clsComment = New CComment With clsComment .AddNameFromCite hLIComment.getElementsByTagName("cite")(0) .AddDate hLIComment.getElementsByClassName("comment-meta commentmetadata")(0) .AddContent hLIComment.getElementsByTagName("p") End With clsComments.Add clsComment Next hLIComment clsComments.CreateSQLFile End Sub |
I looked at the source for the web page to figure out how it was laid out and how to get at the data I needed. The CComment and CComments classes store the data as I loop through the list index items in the comment list. The first CComment method is AddNameFromCite. I didn’t even know there was a Cite tag in HTML (but you could fill a warehouse with what I don’t know about HTML).
|
1 2 3 4 5 6 7 8 9 10 11 12 13 |
Public Sub AddNameFromCite(ByVal hCite As MSHTML.HTMLPhraseElement) Dim hAnchor As MSHTML.HTMLAnchorElement Me.Author = hCite.innerText Set hAnchor = hCite.getElementsByTagName("a")(0) If Not hAnchor Is Nothing Then Me.AuthorLink = Mid$(hAnchor.href, InStr(2, hAnchor.href, "http://"), Len(hAnchor.href)) End If End Sub |
I made this a method because I generally reserve properties to getting/setting values. If I change more than one property or do any extensive manipulation, I go with a method instead of a property. I’m not uber-consistent about it though. The comment author’s name is the innertext of the PhraseElement (that’s what a Cite is, at least according to the TypeName function). To get the AuthorLink, I need to find the anchor and get the href attribute. Because the wayback machine put its own URL in from of other URLs, I had to find the second instance of “http://” to get the real link. Next the AddDate method.
|
1 2 3 4 5 6 7 8 9 10 11 |
Public Sub AddDate(ByVal hDiv As MSHTML.HTMLDivElement) Dim sDate As String Dim vaDate As Variant sDate = hDiv.innerText vaDate = Split(sDate, " at ") Me.CommentDate = DateValue(vaDate(0)) + TimeValue(vaDate(1)) End Sub |
This really should have been a property instead of a method, but oh well. The innertext of the DivElement is something like “January 1, 2010 at 6:16 am”. I split that string on the “at” and used DateValue and TimeValue to build a date. Finally the content of the comment.
|
1 2 3 4 5 6 7 8 9 10 11 12 |
Public Sub AddContent(ByVal hParas As MSHTML.IHTMLElementCollection) Dim hPara As MSHTML.HTMLParaElement Dim sContent As String For Each hPara In hParas sContent = sContent & hPara.innerText Next hPara Me.Content = sContent End Sub |
I passed in a collection of elements that are ParaElements (tag=p=paragraph). Then I looped through them and concatenated a string for the content. By looping through just the p elements, I skip all the comment meta crap that is auto-generated by WordPress and just get to the text.
At this point I have 85 CComment objects and I’m ready to build the SQL string.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 |
Public Sub CreateSQLFile() Dim sFile As String, lFile As Long Dim clsComment As CComment Dim sSql As String Dim aSql() As String Dim lCnt As Long ReDim aSql(1 To Me.Count) 'Build the first part of the sql string with the column names sSql = "INSERT INTO `wp_comments` (`comment_post_ID`, `comment_author`, `comment_author_email`, `comment_author_url`," sSql = sSql & Space(1) & "`comment_author_IP`, `comment_date`, `comment_date_gmt`, `comment_content`, `comment_karma`," sSql = sSql & Space(1) & "`comment_approved`, `comment_agent`, `comment_type`, `comment_parent`, `user_id`) VALUES" & vbNewLine 'Put all the comment values in an array For Each clsComment In Me lCnt = lCnt + 1 aSql(lCnt) = clsComment.SQLInsert Next clsComment 'put it all together sSql = sSql & Join(aSql, ", " & vbNewLine) & ";" 'write it to a sql file sFile = ThisWorkbook.Path & Application.PathSeparator & "wp_incellcomments.sql" lFile = FreeFile Open sFile For Output As lFile Print #lFile, sSql Close lFile End Sub |
Just a bunch string building and putting in a file that I can import into PHPMyAdmin. In the CComment class, the values are put together like this
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 |
Public Property Get SQLInsert() As String Dim sReturn As String Dim aReturn(1 To 14) As Variant Const sNOVALUE As String = "''" Const sSQ As String = "'" aReturn(1) = "7534" aReturn(2) = sSQ & EscSq(Me.Author) & sSQ aReturn(3) = sNOVALUE aReturn(4) = sSQ & EscSq(Me.AuthorLink) & sSQ aReturn(5) = sNOVALUE aReturn(6) = sSQ & Format(Me.CommentDate, "yyyy-mm-dd hh:mm:ss") & sSQ aReturn(7) = aReturn(6) aReturn(8) = sSQ & EscSq(Me.ContentScrubbed) & sSQ aReturn(9) = 0 aReturn(10) = sSQ & "1" & sSQ aReturn(11) = sNOVALUE aReturn(12) = sNOVALUE aReturn(13) = 0 aReturn(14) = 0 sReturn = "(" & Join(aReturn, ", ") & ")" SQLInsert = sReturn End Property |
I really like this method of building a string – putting it into an array and using Join – so I think I’ll start using it. The EscSq function turns any single quotes into two single quotes. The ContentScrubbed property converts any vbNewLines into \r\n. I exported some existing comments from MySQL to see how all this stuff went together. In the end, I ended up with a file that looks like this.
phpMyAdmin kept erroring out that the file was using too much memory. It’s 51kb, so I knew that wasn’t true. But the helpful people at HostGator imported it for me and set me up with console access so I can do it myself next time. I just need to learn the commandline stuff for importing.
I took a quick look through through the comments and they look alright. It’s hard to tell what I screwed up formatting-wise because some people use code tags and most don’t. But the info appears to be there and that’s the most important thing. I guess since I have this set up, I should go back and make sure any other lost posts get their comments too.
As always, if you see something that’s not right on the site, shoot me an email. I have a few hundred posts that still look like crap, but are readable and I’m fixing them as I see them.
I’ve wanted to have some wiki-like diffing in my userform textboxes for a while now. Since I’ve been using wikis almost daily, I want the revisioning feature in everything I do. I’m not there yet, but I decided to see what kind of algorithm I would need to do it. I read the Wikipedia article on longest common subsequence and played around with it a little.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 |
Public Function LCSTable(ByRef aOriginal() As String, ByRef aRevised() As String) As Variant Dim aReturn() As Long Dim i As Long, j As Long 'aOriginal and aRevised should be 1-based. Here we make a matrix with a gutter 'row and column that will always be zero. ReDim aReturn(0 To UBound(aOriginal), 0 To UBound(aRevised)) For i = 1 To UBound(aOriginal) For j = 1 To UBound(aRevised) 'If the elements match, bump up the count from the element 'one up and one left If aOriginal(i) = aRevised(j) Then aReturn(i, j) = aReturn(i - 1, j - 1) + 1 Else 'If they don't match, copy the largest from either above or from the left aReturn(i, j) = Application.WorksheetFunction.Max(aReturn(i, j - 1), aReturn(i - 1, j)) End If Next j Next i LCSTable = aReturn End Function |
This code is called LCSLength in the article. It returns a matrix (2d array) with counts of matching elements at each position. For instance, if you’re diffing “Dick” and “Rick”, they have three letters in common and this table will compute that. It looks like this
| R | i | c | k | ||
| 0 | 0 | 0 | 0 | 0 | |
| D | 0 | 0 | 0 | 0 | 0 |
| i | 0 | 0 | 1 | 1 | 1 |
| c | 0 | 0 | 1 | 2 | 2 |
| k | 0 | 0 | 1 | 2 | 3 |
The rest of the functions use this table to figure out what’s what.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 |
Public Function LCSString(ByRef vaTable As Variant, ByRef aOriginal() As String, ByRef aRevised() As String, ByVal i As Long, ByVal j As Long) As String Dim sReturn As String If i = 0 Or j = 0 Then sReturn = "" ElseIf aOriginal(i) = aRevised(j) Then sReturn = LCSString(vaTable, aOriginal, aRevised, i - 1, j - 1) & aOriginal(i) Else If vaTable(i, j - 1) > vaTable(i - 1, j) Then sReturn = LCSString(vaTable, aOriginal, aRevised, i, j - 1) Else sReturn = LCSString(vaTable, aOriginal, aRevised, i - 1, j) End If End If LCSString = sReturn End Function |
This function (called backtrack in the article) traces back through the table and outputs the longest common subsequence. It’s a recursive function (it calls itself) and continually appends letters (or other elements) on to the return string.
When both the i and j counters are zero, it stops calling itself. Otherwise, if the two letters match, it appends the current letter to the end and calls itself using the element up and to the left. If there’s no match, it goes to the larger of the element above (i-1) and the one to the left (j-1). By following the path of the larger numbers through the matrix, it can find the common letters. It’s originally called with the largest i and j – in the above table, it’s called looking at the 3 (the bottom right cell). Here’s how it tracks through the matrix (I’ll use cell references, but it’s not really cells).
Thrilling, isn’t it?
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 |
Public Sub PrintDiff(ByRef vaTable As Variant, ByRef aOriginal() As String, ByRef aRevised() As String, ByVal i As Long, ByVal j As Long) If i > 0 Or j > 0 Then If i = 0 Then PrintDiff vaTable, aOriginal, aRevised, i, j - 1 Debug.Print "+" & Space(1) & aRevised(j) ElseIf j = 0 Then PrintDiff vaTable, aOriginal, aRevised, i - 1, j Debug.Print "-" & Space(1) & aOriginal(i) Else If aOriginal(i) = aRevised(j) Then PrintDiff vaTable, aOriginal, aRevised, i - 1, j - 1 Debug.Print Space(2) & aOriginal(i) ElseIf vaTable(i, j - 1) >= vaTable(i - 1, j) Then PrintDiff vaTable, aOriginal, aRevised, i, j - 1 Debug.Print "+" & Space(1) & aRevised(j) ElseIf vaTable(i, j - 1) < vaTable(i - 1, j) Then PrintDiff vaTable, aOriginal, aRevised, i - 1, j Debug.Print "-" & Space(1) & aOriginal(i) Else Debug.Print End If End If End If End Sub |
This is another recursive function working backward through the matrix. When it finds a match, there’s no prefix. If it’s a new element (in Revised, but not Original) the prefix is a “+”. If it’s a deleted element, you get a “-“. This prints the results to the immediate window. Let’s look at some examples.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 |
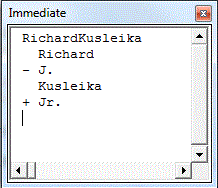
Public Sub DiffLetters() Dim sOriginal As String Dim sRevised As String Dim vaTable As Variant Dim i As Long Dim aOriginal() As String Dim aRevised() As String 'Create strings sOriginal = "Richard J. Kusleika" sRevised = "Richard Kusleika Jr." 'Make an array of letters ReDim aOriginal(1 To Len(sOriginal)) For i = 1 To Len(sOriginal) aOriginal(i) = Mid$(sOriginal, i, 1) Next i ReDim aRevised(1 To Len(sRevised)) For i = 1 To Len(sRevised) aRevised(i) = Mid$(sRevised, i, 1) Next i 'Create the longest common sequence matrix vaTable = LCSTable(aOriginal, aRevised) 'Print the longest common sequence Debug.Print LCSString(vaTable, aOriginal, aRevised, UBound(aOriginal), UBound(aRevised)) 'Show the diff between the letters PrintDiff vaTable, aOriginal, aRevised, UBound(aOriginal), UBound(aRevised) End Sub |
This shows the diff on a letter-by-letter basis.
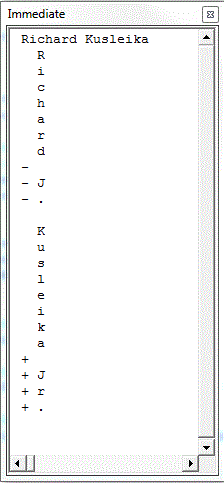
The first line is a printout of the longest common subsequence. The rest is a letter-by-letter diff that shows which elements were added, deleted, and unchanged. We can also diff on words.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 |
Public Sub DiffWords() Dim sOriginal As String Dim sRevised As String Dim aOriginal() As String Dim aRevised() As String Dim vaTable As Variant sOriginal = "Richard J. Kusleika" sRevised = "Richard Kusleika Jr." aOriginal = Split(Space(1) & sOriginal, Space(1)) aRevised = Split(Space(1) & sRevised, Space(1)) vaTable = LCSTable(aOriginal, aRevised) Debug.Print LCSString(vaTable, aOriginal, aRevised, UBound(aOriginal), UBound(aRevised)) PrintDiff vaTable, aOriginal, aRevised, UBound(aOriginal), UBound(aRevised) End Sub |
Instead of filling the array with letters, I split the string on spaces to get words. Note that I put a leading space in front of each string before the split. The array needs to be 1-based and the Split function is zero based. The array doesn’t actually need to be 1-based, but the first row and column is ignored, so I made sure that it was something I didn’t care about. Once the arrays are filled, everything is the same.
Traditionally, diffing text is done line-by-line. So let’s do that. I found an example essay and made two files; OriginalDiff.txt and RevisedDiff.txt. I changed one thing in Revised and used this code to diff them.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 |
Public Sub DiffLines() Dim sFile As String Dim lFile As Long Dim aOriginal() As String Dim aRevised() As String Dim lCnt As Long Dim vaTable As Variant sFile = Environ$("USERPROFILE") & "\Dropbox\Excel\OrignalDiff.txt" lFile = FreeFile Open sFile For Input As lFile Do While Not EOF(lFile) lCnt = lCnt + 1 ReDim Preserve aOriginal(1 To lCnt) Line Input #lFile, aOriginal(lCnt) Loop Close lFile sFile = Environ$("USERPROFILE") & "\Dropbox\Excel\RevisedDiff.txt" lCnt = 0 Open sFile For Input As lFile Do While Not EOF(lFile) lCnt = lCnt + 1 ReDim Preserve aRevised(1 To lCnt) Line Input #lFile, aRevised(lCnt) Loop Close lFile vaTable = LCSTable(aOriginal, aRevised) PrintDiff vaTable, aOriginal, aRevised, UBound(aOriginal), UBound(aRevised) End Sub |
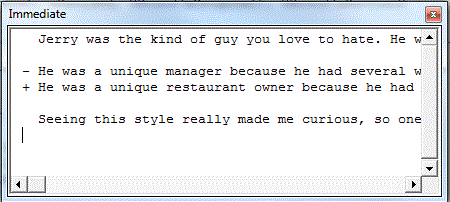
And that’s as far as I got. Next, I need to put the diffs into a database so I can display diffs and revert to prior versions. Or, quite possibly, I’ll lose interest because I don’t have a burning need for this. It’s just something I’ve wanted to do.
You can download Diffing.zip